Estimating Forest Sustainability Bond Prices for Natural Resource and Ecosystem Services Markets

4 years ago

4,276

While forest and “green” bonds are traded today, our findings demonstrate that the return from an integrated portfolio that contains forest wood products (direct use)…

Richard L. Bernknopf
Research Professor of Economics, The University of New Mexico

Craig D. Broadbent
Professor of Economics, Brigham Young University-Idaho

Abstract

Existing markets for natural resources commonly trade precious metals, energy, and minerals. More recently, ecosystem service markets are being developed, including the resources of land carbon, species habitats, streams, watersheds, and wetlands. We introduce a sustainable forestry bond that is composed of wood products and ecosystem services. The security represents a specific forested land quality and quantity for the production of natural resources and ecosystem services. An investment decision is based on the Net Resource Value (NRV) of a series of cash flows produced from trees and benefits provisioned by the ecosystem services in a forest. This combination of inputs and outputs represents the value created over the lifetime of a forest project and is equal to the monetized difference between the forest natural resources and ecosystem service outputs and the capital invested to produce them. For investment decision making, an Average Internal Rate of Return or AIRR is calculated, which is Net Present Value-consistent (NPV-consistent).

A significant finding of adding all these ecosystem services is that they produce an increase in the cash flow to a traditional forest bond, which, in turn affects the security’s Par value, and consequently the Return on Investment. Our results demonstrate that reforestation with a multiple harvest species maximizes direct-use benefits and provisions significant carbon sequestration benefits. However, land conversion to such a species can have long-term environmental effects that fundamentally change the structure of an ecosystem. To account for these environmental changes, we include two other ecosystem endpoints in the portfolio (i.e., waterfowl habitat and nitrate reduction). While forest and “green” bonds are traded today, our findings demonstrate that the return from an integrated portfolio that contains forest wood products (direct use) and ecosystem services (indirect uses) provides an investor with more investment choices.

I. Introduction: Estimating Forest Sustainability Bond Prices for Natural Resource and Ecosystem Services Markets

There is ample evidence of investor interest in forests, which provide a means to diversify financial investment portfolios and to securitize sustainable forests with green bonds (Erhart 2018; ECLAC 2017). For years, forest bonds have been used as a means to diversify an investment portfolio (Healy et al. 2005). On the other hand, green bonds for environmental sustainability, by preserving and developing forests, are a recent development (ECLAC, 2017; Madeira, 2016). Of the USD 6.5 billion invested toward capital during the years 2004–2015, investors directed 44% (USD 2.9 billion) of that capital toward sustainable forestry (Hamrick 2016). There are several green exchanges globally (Erhart 2018). In addition, there are evolving partnerships and quasi-markets in the United States. For example, the U.S. Department of Agriculture (USDA) supports conservation programs designed to sustain or restore ecosystem services. These market-oriented conservation programs have increased in value from USD 0.5 billion in 1986 to just less than USD 5 billion annually since 2015. In An Atlas of Ecosystem Markets in the United States, Forest Trends’ Ecosystem Marketplace reported that at least USD 2.8 billion is transacted every year through ecosystem markets in the United States (Bennett et al., 2016). The largest markets include land carbon, species habitats, streams, watersheds, and wetlands in many forms (Pindilli and Casey 2015). Carbon markets also exist at the state, regional, and international levels (Goulder 2013; Burtraw 2016).

There are applications of portfolio theory to estimate the ROI and risks involved in ecosystem services management (Boyd and Epanchin-Niell 2017; Sanchirico et al. 2008; Benitez et al. 2007; Benitez and Obersteiner 2006; Edwards et al. 2004). These examples demonstrate how a risk-return decision framework involves estimation of the economic return to a market commodity such as fish (Sanchirico et al. 2008) and the risk to an ecosystem service such as deforestation (Benitez et al. 2007).

Ecosystem processes and services are known to be interdependent at different spatial and temporal scales and can be positively or negatively correlated. Ecology, economics, and geography literatures acknowledge these interdependencies. Holling and Gunderson (2002) discuss interdependence between fast and slow ecological processes and the effects of human intervention on those processes. Geographers such as C. Raudsepp-Hearne et al. (2010) describe how ecosystem service bundles can have negative or positive effects on each other within an ecosystem. Ingram and Malamud-Roam (2015) explore the interdependence of short and long climate cycles on biophysical processes. Lastly, Boyd and Banzhaf (2007) define and describe the physical and economic processes that lead to ecosystem endpoints. Ecosystem endpoints have value to humans and can be considered end-use commodities in an economic framework. All of the above authors agree that ecosystem services are interdependent.

Although there are examples of direct-use returns (grayish) in conventional forest funds or indirect-use returns (greenish) for ecosystem services provisioned by forests, such as carbon sequestered and water quality and quantity in a watershed, trading occurs in separate markets. It is likely that wood products and ecosystem services occur in the same forest and similarly increase in value over time, which means they could be integrated into one security for public markets. A public market security listing on an exchange can be a combination of both assets that is based on the weighted sum of a traditional bond and a green bond for the same land (Bass et al. 2019). This approach for a sustainable forestry bond has two effects. First, it increases the yield of a traditional forest bond by including the return on investment from the green portion of asset value. Second, calculation of cash flows need only to account for investment and operating costs once rather than twice if the bonds were traded in separate markets or exchanges.

Market value is created from two component portfolios, each of which has its own rate of return and risk. Value creation for both wood products and ecosystem services have a correlation close to 1.0, whereas the correlation among ecosystem services can be negative or positive. The portfolio model developed here exploits this information to form a sustainable forestry bond. In addition to marketed products, a sustainable forestry bond should contain a second set of assets, namely ecosystem services such as waterfowl habitat, nitrate retention and carbon sequestration.

We develop the metrics for estimating the Average Internal Rate of Return (AIRR) and the current market price of a sustainable forestry bond of wood products (direct use) and multiple ecosystem services (indirect uses). Cash flows are estimated with and without a timber harvest, and with and without ecosystem services with land and forest management costs. Correlations among resources are estimated, which affect the outputs of ecosystem services depending on the land cover and existing land use (e.g., bottomland agriculture). Market value is created from two component portfolios, each of which has its own rate of return and risk. An investment decision is based on whether the AIRR of the forest exceeds the market or a required rate of return.

The example includes two types of forests and three ecosystem services in Tensas and Madison Parishes, Louisiana. Market (or appraised) values are employed for wood products and land and management costs and appraised values are estimated for ecosystem services. We assume an investor’s objective is to maximize portfolio return or to minimize portfolio risk given a greenness preference. Application of alternative combinations of forest assets (treatments) that arise in the example can influence the investment choice in a market exchange. An investor has an initial amount of wealth to be invested in each of the two portfolios that, when combined, result in the sustainable forestry bond AIRR.

The paper is organized as follows: section II contains a derivation of the value created from a managed forest and is applied to a sustainable forestry bond investment decision (Marchioni and Magni 2018). Section III contains an application of a portfolio model for producing forest direct uses and provisioning indirect uses in the sustainable forestry bond. Section IV presents the results of the model application in two parishes in northern Louisiana (167,745 hectares), and section V is a discussion of the model, application, and avenues for future research.

II. A Sustainable Forestry Bond and Portfolio Opportunity Set

A publicly tradeable security in the form of a sustainable forestry bond is created based on the conversion of land to forests with the highest expected average return on investment (direct- and indirect-use returns) at time $t$ minus the expected opportunity cost of undertaking that conversion (Lubowski et al. 2008). Land in use $l,\ l=1,\ldots,L$ will be reallocated use $j,\ j\neq\ l,$ if it maximizes the economic return after conversion costs that are greater than the return to $l$ ; otherwise the land will remain in its current use at time $t$ .

An investment decision is based on the net present value $(NPV)$ of a series of cash flows produced from trees $(\nu)$ and benefits $(b)$ provisioned by the ecosystem services in a forest $(F)$ at a discount rate $(r)$ . This combination of inputs and outputs represents the value created over the lifetime of the forest project and can be evaluated by calculating its $NPV$ . By adding ecosystem services as an asset, we create a new component of value for the forest (Fenichel and Abbott, 2014). The value of the ecosystem services affects the forest $NPV$ positively, which is relevant to an investment decision. Since we combine direct- and indirect-use assets, we label the combination a sustainable forestry bond to have a net resource value $(NRV)$ . The $NRV$ is equal to the monetized difference between the forest natural resources and ecosystem service outputs and the capital invested to produce them. For investment decision making, the $NRV$ is used to estimate an AIRR $\left(\bar{r}\right)$ , which is NPV-consistent (Magni, 2010).

A land asset scenario $s$ produces an $NRV$ for forest $F$ . Each $s,\ s=1,\ldots,S$ forest scenarios, produce direct use benefits ( $bν$ ) and indirect use ecosystems services ( $bi$ ) that are estimated via production functions for a land assessment unit $k$ (Heal et al.2005). The addition of ecosystem services as a land asset builds on the idea that a quantity change is a sustainability dividend (Bond et al. 2017). Quantities ${\ q}_\nu$ and $q_i$ are market goods produced and ecosystem services provisioned, respectively. The forest outputs have benefits and risks that arise from, among other things, colocation[1]. A market value for direct-use products $b_v,\ \upsilon=1,\ldots,\ \Upsilon$ and an indirect-use value $b_i,\ i=1,\ldots,B$ interdependent ecosystem services generate a monetized value for the two components of a scenario $s$ .

[1]. Environmental risks such as wildfires occur at a low rate of approximately 1% annually. The majority of timber to a fire often can be salvaged (Healy et al. 2005).

The expected cash flows derived from wood and ecosystem service products and risks associated with the tradeoff between the assets in the portfolios. Estimation of the expected value $\mathbb{E}\left(\upsilon_k\right)$ , for the direct-use asset depends on the land characteristics of location $k$ for commodities revenue $\upsilon_k,\ \upsilon_k=0,\ldots\ \Upsilon_k$ . Marketable commodities are a function of current prices and the quantity of the renewable resource harvested.

$\mathbb{E}\left(\upsilon_k\right)=p_{s\upsilon}\left(-{cp}_k+b_\nu\cdot\left(1+r_{\nu_n}\right)^{{RI}_k}\right)$ (1)

where $\mathbb{E}\left(\upsilon_k\right)$ is the expected $NPV$ for direct use of (a) rotation(s) of production, $p_{s\upsilon}$ is the probability of timber harvested for scenario $s$ , $RIk$ is a land use rotation interval of $t$ years, $t=0,\ldots,n,$ for $k$ grid cells in a forest, $k=1,\ldots,K$ , ${cp}_k$ are the land, investment and management costs for $k$ grid cells, $b_\nu={pw}_k\cdot\ q_{V_k}$ , $b_\nu$ is the economic benefit from direct-use production, ${pw}_k$ is the direct-use price for $k$ grid cells, $q_{V_k}$ is quantity produced, and $r_{\nu_n}$ is the market discount rate.

Estimation of the expected value $\mathbb{E}\left(B_k\right),$ for the indirect-use asset also depends on the land characteristics of location $k$ and the quantity of the renewable resource harvested. In addition, ecosystem services possess interdependencies that can be positively or negatively correlated in the $k$ th cell of land. The spatial correlations affect the qualities and quantities of ecosystem services that are provisioned ultimately. Because a land assessment unit provisions a variety of ecosystem services the security is the weighted average of the expected benefits for the indirect use:

$\mathbb{E}\left(B_k\right)=p_{sb_i}\left(\sum_{k=1}^{K}\sum_{i=0}^{I}\left(b_{ik}\right)\bullet\left(\left(1+r_{B_n}\right)^{{RI}_k}\right)\right)$ (2)

where $\mathbb{E}\left(B_k\right)$ is the expected forest $NPV$ of the interdependent ecosystem service benefits in $k$ for $RIk$ land use rotation(s) in $F$ , $B_k$ is an estimate of the gross conservation return (Murdoch et al. 2007), $r_{B_n}$ is a green / social rate of discount, $p_{sb_i}$ is the joint probability where $p_{sb_i}=p_s\cdot p\left(b_i\left|s\right.\right), p\left(b_i\left|s\right.\right)$ is the conditional probability of obtaining a quantity provisioned for ecosystem service $i$ given scenario $s$ , and $\left(b_{ik}\right)$ is the expected benefit from ecosystem service $i$ in $k$ in $F$ . The appendix contains the method for calculating ecosystem service provision benefits. When treated as an asset for a forest harvest rotation time period, ${RI}_k=n$ , or the forest can remain intact in perpetuity, and ${RI}_k=\infty$ . By adding ecosystem services provisioned in equation 2 to direct-use benefits in equation 1, the new combined value is denoted as the forest Net Resource Value ${NRV}_F$ :

${NRV}_F\equiv\mathbb{E}\left(\upsilon_k\right)+\mathbb{E}\left(B_k\right)$ (3)

NRV like Net Present Value can be considered a reliable tool for investment decisions, since it correctly measures value creation (Marchioni and Magni 2018). Application of the AIRR to the project, according to Marchioni and Magni (2018), is defined as the ratio of the overall returnearned by the investor to the overall capital committed by the investor, or as the weighted mean of period rates associated with the capital stream:

$\bar{r}=r^\ast+((\frac{\sum_{t=1}^{n}{\iota_t{{cp}_k}_{t-1}}d_{t-1}}{\sum_{t=1}^{n}{{cp}_k}_{t-1}d_{t-1}})-1)\bullet\left(1+r^\ast\right)$ (4)

where $\bar{r}$ is the average internal rate of return, ${cp}_k=c_0+\sum_{t=0}^{n}\frac{c_t}{\left(1+r\right)^t},$ $c_0$ is land and other initial investment, and $c_t$ are operating and management costs for $k$ grid cells, and $c_n=0.$ $r_t$ is the return in $t$ , $r_t=\sfrac{{NRV}_t}{{{cp}_k}_{t-1}},$ and $d_t=\left(1+r\right)^{-t}$ . The security AIRR has a direct use ${\bar{r}}_{\upsilon\left(s\right)}$ , and an indirect use ${\bar{r}}_{B\left(s\right)}$ . The AIRR is $NPV$ -consistent and leads to the decision rule $\varphi$ :

An investment project creates value if and only if $\varphi>r$ (Marchioni and Magni 2018).

If $\varphi=\bar{r}$ , the following relationship exists:

${NRV}_F\left(1+r\right)={cp}_k\left(\bar{r}-r\right)$ (5)

The decision rule and equation (5) can be reworked to be in terms of a capital base ${cp}_k$ and an excess return can be estimated as:

$\bar{r}-r>0$ (6)

Estimation of $\bar{r}$ assumes that the investment is made in the initial year and remains invested for the project period. The period return rate is ${ROI}_t=\frac{\mathrm{Operating\ Profit}\ }{\mathrm{Investment\ Cost}}$ and the AIRR for an investment scenario ${\bar{r}}_s$ is (Marchioni and Magni 2018):

${\bar{r}}_s=r^\ast+((\frac{\sum_{t=1}^{n}{\sum_{k=1}^{K}\left(\upsilon_{k_t}+B_{k_t}\right)\bullet d_{t-1}}}{\sum_{t=0}^{n}\frac{c_{n-1}}{\left(1+r\right)^{n-1}}})-1)\bullet\left(1+r^\ast\right)$ (7)

It is assumed that ${\bar{r}}_s$ is distributed normally (Laffont 1989).

We apply the AIRR over the $S$ scenarios of multiple assets (wood and ecosystem services) for a range of treatments to populate a portfolio opportunity set. Treatments allow us to conduct sensitivity analysis and estimate the variance among the scenarios (e.g., the two components of the security can have different or equal AIRR’s and discount rates). The objective of the investor is to maximize ${\bar{r}}_s$ , which is a reliable measure of worth.

Security risk is a lack of perfect knowledge about inputs (e.g., resources, technology, regulations) and outcomes (e.g. revenues, fire disturbance, and ecosystem changes) for a time in the future. To measure the risk, we estimate the standard deviations for the direct-use component in a manner consistent with existing portfolio theory (Sharpe et al., 1999; Cubbage et al., 2014; Mei and Clutter 2010) and estimate the standard deviation for the indirect-use component from equation 8:

$\sigma_{B_s}=\sqrt{\sigma_{b_{ik}}^2w_{b_{ik}}^2+\sigma_{b_{jk}}^2w_{b_{jk}}^2+2w_{b_{ik}}w_{b_{\zeta k}}{COV}_{b_{i\zeta k}}}\ ,\ \ \ \ \ k=1,\ldots,K$ (8)

where $w_{b_{ik}}$ is the proportion (weight) of investment in ecosystem service $i$ , $w_{b_{\zeta k}}$ is the proportion (weight) of investment in ecosystem $\zeta, {COV}_{b_{i\zeta k}}$ is the covariance of returns between ecosystem service $i$ and $\zeta$ . The covariance is expressed as ${COV}_{b_{i\zeta k}}=\rho_{b_{i\zeta k}}\sigma_{ik}\sigma_{\zeta k}$ , where $\rho_{b_{i\zeta k}}$ is the correlation between ecosystem service $i$ , and ecosystem service $\zeta\Biggm$ [2]. The covariance of the services in a scenario is important for understanding the interaction among services. A negative covariance is a reduction in scenario variance because expected returns move in opposite directions. This type of correlation is associated with a diversified scenario. On the other hand, assuming a positive covariance among ecosystem services would amplify a scenario standard deviation.

[2]. $\rho_{b_{i\zeta k}}=\sum_{i,\zeta}{p_{b_{ik}b_{\zeta k}}\left(\frac{b_{ik}-\mathbb{E}\left(b_{ik}\right)}{\sigma_{ik}}\right)}\left(\frac{b_{\zeta k}-\mathbb{E}\left(b_{\zeta k}\right)}{\sigma_{\zeta k}}\right)$ , where $i\neq\zeta,\ i=1,\ldots,\ I.\ \zeta=1,\ldots,\ I, p_{b_{ik}b_{\zeta k}}$ is the joint probability of $b_{ik}$ and ${\ b}_{\zeta k}$ in $k$ cells, and $\sigma_{ik}$ and $\sigma_{\zeta k}$ are the standard deviations for ecosystem services $i$ and $\zeta$ respectively.

Like the sustainable forestry bond, portfolio construction is based completely on the AIRR and the standard deviation of the AIRR of the bond portfolio. In the portfolio analysis, the $S$ scenarios are the investment choices for comparison to an existing baseline land use, such as bottomland agriculture (Boyd et al. 2015). The AIRR for a range of forest treatments is ${\bar{r}}_s$ composed of ${\bar{r}}_{\upsilon_s}$ and ${\bar{r}}_{B_s}$ divided by the investment cost. Depending on the investor’s objectives for ${\bar{r}}_s$ and $\sigma_s^2$ , the securities could be oriented more (less) toward market goods and less (more) toward provisioning of ecosystem services.

A key aspect of portfolio theory is the role of the statistical correlation between the direct- and indirect-use portfolios. The two assets are correlated because the ecosystem service benefits are a known externality affected by the market good. In a renewable resource case, a forest of trees produces timber as well as ecosystem services and are expected to have a positive correlation[3]. The correlations enter the analysis in the calculation of bond and portfolio risks. Each asset group is itself a portfolio and becomes an input to the opportunity set (Sigman 2005). The exception is NRV₀, the baseline, and is not included in the opportunity set. Thus, the risk is the variance of the direct use and indirect use provisioned, respectively:

$\sigma_s^2=f^2\left(\sigma_\upsilon\right)^2+\left(1-f\right)^2\left(\sigma_B\right)^2+2f\left(1-f\right)\sigma_\upsilon\sigma_B\rho_{\upsilon B}$ (9)

where $\sigma_\upsilon$ and $\sigma_B$ are the standard deviations of the stochastic returns to direct-use production and ecosystem service provision of a scenario, and $\rho_{\upsilon B}$ is the correlation between direct-use production and ecosystem service provision. We consider a case of applying the spatial portfolio to assess the return of managed forest land. The portfolio contains timber and other wood products and ecosystem services via reforestation in Louisiana.

[3.] In a nonrenewable resource example, (e.g., fossil fuels) extraction and changes to ecosystem services are expected to have a negative correlation.

III. Application: Sustainable Forestry Bond Pricing

In this section, a variety of managed forests AIRR’s are estimated for pricing a long-term sustainable forestry bond. The type of forest and its location will affect the intrinsic value of the investment for timber and ecosystem services. The process is for the investor to judge among scenarios of forest opportunities that maximizes ${\bar{r}}_s$ . We compare a one species versus a multispecies forest as a 30-year sustainable forestry bond. Below, ${\bar{r}}_s$ and a current market price for a zero-coupon[4] and a traditional bond[5] .

[4]. A zero-coupon bond is a bond that (1) pays no interest but instead is sold at a deep discount on its par-value, or (2) an interest paying bond that has been stripped of its coupon which is sold separately as a security in its own right. Bondholder’s income is determined by the difference between the bond’s redemption value on maturity and its purchase price (Business Dictionary 2020a).

[5]. A traditional bond is a bond issued with a number of coupons that must be presented to receive the periodic interest (Business Dictionary 2020b).

Demand for forest lands has been driven by changes to forest and timber market institutions, environmental policies and regulations, and incentives and tax measures for pension plans (Mei and Clutter 2010; Yao et al. 2016). Policies such as the Employee Retirement Income Security Act of 1974 have incentivized forestation (Mei and Clutter 2010; Binkley 2004). One outcome of the legislation is that forests and forest shares have become a growing part of land investment portfolios (Mei and Clutter 2010). The forest type will affect the terminal value of a forest investment security (Bass et al. 2019; Redmond and Cubbage 1988).

The investment is a multi-year decision. Alternatives may range between high production of wood products with multiple harvest cycles and an infinite planning horizon for preservation and no harvest. Although there have been references of the need to quantify the environmental benefits in this type of investment, they have been considered an externality (Rinaldi and Jonsson 2013; Cubbage et al. 2014; Frey et al. 2013; Binkley et al. 2006; Washburn and Binkley 1989). Only recently have green bonds and impact investing become available to public markets (Bass et al. 2019; Bennett et al. 2016). Although a variety of funds exist, the investment returns are dependent on various levels of quantification for generating revenues. Strategies include sales of timber, carbon offsets, other forest products, and land rights for permanent conservation (e.g., easements); and land leasing (Bass et al. 2019). But no method to estimate the within asset correlations among the indirect uses has been included.

A. Forest Production

Location affects development and management efforts. Location quality is based on the size, age, condition, and configuration of Land Use and Land Cover (LULC) patches and can be represented as a distribution over the total number of these patches (Chivoiu et al. 2010). To be useful and traded in a market the areal unit must capture these landscape heterogeneities (Convertino and Valverde 2013).

Currently, a forest Return on Investment is based on the terminal value of the timber and other wood products (Cascio and Clutter 2008; Healy et al. 2005; Washburn and Binkley 1993; Redmond and Cubbage 1988). Investment analysis compares the asset (timber and wood products) value to a market or required rate of return $r_F^\ast,$ (Cascio and Clutter 2008; Sun and Zhang 2001). In a market of this type, environmental assets are considered positive environmental externalities (Herbohn and Henderson 2002).

For timber, the forest yield in timber products is the stumpage price times the amount of wood removed (Straka et al. 2001). Two key elements affect the timber yield: site index and stocking. The site index describes the quality of land for growing trees (soil productivity). Site refers to a tree species; for example, pine or oak sites. Few species grow equally well on the same site. In our application, forest production estimates are for Eucalyptus and mixed hardwood trees. The capital gain is the result of the accumulation of the volume and quality of the trees (stock) that is distributed as a cash flow to investors (Healy et al. 2005). The quantity produced can be projected via a production function (Van Kooten and Bulte 2000). The yield of the forest is based on the annual cash flows over a period of years to a planned time horizon for harvest.

Ecosystem services are recognized as non-timber forest outputs. Designation of what is a direct or indirect use is defined by the Food and Agricultural Organization of the UN (Lange 2004). Estimation of the benefits of the ecosystem services is based on landscape qualities that have a range of biophysical characteristics and corresponding ecosystem services. The management scenarios and treatments provision the same three ecosystem services: carbon sequestration, nitrate retention, and enhanced habitat for waterfowl. However, the proportion of the benefits invested in each service varies.

B. Sustainable Forestry Bond Estimation

Securitization for possible reforestation of forests in Tensas and Madison LA parishes in the Lower Mississippi Valley (LMV) is the region considered for the example. For accounting purposes, the forested lands are described within hydrologic units (Seaber et al. 1994). Hydrologic unit codes provide a geographic and hydrologic framework for water resources planning and include drainage, hydrography, culture, political and hydrologic unit boundaries. Analysis is conducted for reforestation of bottomland forests hydrologic units[6] in the LMV.

[6]. The hydrologic unit code is an eight-digit number that identifies each hydrologic unit. The code uniquely identifies each of the four levels-regional, subregional, accounting, and cataloging-of hydrologic classification within four two-digit fields (USGS 1999).

The LMV has experienced extensive loss and conversion of forests and wetlands in the past. The predominant land use is agriculture (the baseline), with pockets of bottomland hardwood forests (Barnett et al. 2016; Faulkner et al. 2011). Bottomland hardwood forests are forested lowland areas along streams and rivers that occur on alluvial floodplains (Barnett et al. 2016). The forested wetlands contained in Tensas and Madison Parishes provision flood attenuation, nutrient filtration, sediment retention, food production, groundwater recharge, wildlife habitat, carbon sequestration, recreational opportunities, cultural and aesthetic values.

The NRV is based on the stock and attributes of trees as well as the co-benefit service flows of the ecosystem services. Capital appreciation represents the change in the market price of the forest assets derived from the benefits of timber and ecosystem services. With the addition of an ecosystem services asset, an investor should expect an increase in the yield of the bond.

Equation 1 provides an estimate of the NPV of the timber and other wood production for one land-use rotation that is then used to determine ${\bar{r}}_{\upsilon_s}$ and $\sigma_{\upsilon_s}$ for Madison and Tensas Parishes. Equation 2 provides the indirect-use component, ${\bar{r}}_{B_s}$ and $\sigma_{B_s}$ for Madison and Tensas Parishes. The expected benefits are based on local values for carbon sequestration ( $carbon$ ), waterfowl abundance ( $wf$ ), and nitrate retention ( $Nitrate$ ). In the indirect-use component, ecosystem services can be negative or positively correlated ranging between -1 and +1.

The market price of the production of the forest includes cash flows for direct, indirect or both uses (Sharpe et al. 1999):

$P_{F\left(s\right)}=\sum_{t=0}^{T}\frac{{NRV}_{F\left(t\right)}}{\left(1+{\bar{r}}_s\right)^t}$ (10)

where $P_{F\left(s\right)}$ is the current market price of a sustainable forestry bond for scenario $s$ . The portfolio risk is measured by the standard deviation of the returns $\sigma_s$ , which is the combined standard deviation of the wood products $\sigma_\upsilon$ and, standard deviation of the ecosystem services $\sigma_B$ .

Traditional forest valuation approaches assume $\mathbb{E}\left(B_K\right)=0$ , so that the capital value and AIRR are derived from direct uses and compared to a required rate of return $r_F^\ast$ for an investment decision. On the other hand, if $\mathbb{E}\left(B_k\right)>0$ , $\upsilon$ $k$ is altered by adding the indirect-use benefits to the security at no extra investment or operating cost. The addition of $Bk$ to the forest security will increase the cash flow and rate of return, and consequently should alter the current security price. On the other hand, investment and operating costs are incurred for the indirect-use component for a forest with an infinite rotation period and no harvest.

The scenarios proposed for investment are available as two distinct types of bonds. First a zero-coupon bond is calculated. All bonds in this category should sell below par if the interest rate is greater than zero. Second, a traditional bond is calculated, which differs from a zero-coupon because the price of the bond depends upon the bond return and its annual coupon. $P_{F\left(s\right)}$ for a forest zero-coupon bond is:

$P_{F\left(s\right)}=\frac{{NRV}_{F\left(T\right)}}{\left(1+{\bar{r}}_s\right)^T}$ (11)

where ${NRV}_{F\left(T\right)}$ is the capital value at maturity (par value) for $t,\ t=\left(1,\ldots,T\right)$ , time periods. Alternatively, the $P_{F\left(s\right)}$ for a traditional bond with a required rate of return $r_F^\ast$ is:

$P_{F\left(s\right)}={\bar{r}}_s\bullet\frac{1-\frac{1}{\left(1+r_F^\ast\right)^n}}{r_F^\ast}+\frac{P_n}{\left(1+r_F^\ast\right)^n}$ , (12)

where ${\bar{r}}_s$ is the coupon rate for timber and other wood products and / or ecosystem services.

C. An Example: Sustainable Forestry Bond

The LMV has had large-scale conservation efforts that have targeted the restoration and enhancement of ecosystem structure, functions, and services (Barnett et al. 2016; Chiviou et al. 2010; Lower Mississippi Valley Joint Venture 2002). These efforts require patch- and landscape-level management, assessment, and monitoring to estimate the effects of changes in LULC. The study region is comprised of agriculture, restored riparian forest (mostly Wetland Reserve Program patches), water features, and mature bottomland hardwood forest. Concentrations of sediments and nutrients were obtained from USGS gage 07369500 within the Tensas River Basin, LA. The input parameter values for sediment and nutrient concentration are average values of available data from the period 01/01/1974 to 12/31/1999.

The analysis is undertaken with a data set that is constructed for the hypothetical example. Trees accumulate woody material (branches, bole, roots) over many years, a layer per period or year (Florida Forest Stewardship 2010). Growth is measured as the change in tree characteristics (weight, basal area, volume, etc.) over a specified amount of time. Different species gain value at different growth rates. For the purpose of exposition the direct-use component required rate of return is based on calculations from Cascio and Clutter (2008). Ecosystem service non-timber forest output quantities are estimated in an empirical model and Decision Support System (Kirilenko et al. 2007). The planning horizon in the example is 100 years for Madison and Tensas Parishes and covers 167,745 hectares in the Tensas River Basin. The hypothetical scenarios are as follows:

Forest scenario NRV₀: The baseline assumes no change in current LULC. It provides ecosystem services provisioned without new investment in forests and is the baseline compared to the managed forest alternatives.

Forest scenario NRV₁: Land conversion of 15% of current agricultural land split between the two parishes to fast growing Eucalyptus species. Trees are harvested every 12 years for biomass energy.

Forest scenario NRV₂: Land conversion of 15% of current agricultural land split between the two parishes to bottomland mixed forest with preference for landscape locations and hydrologic connectivity. Targeting variables include hydric soils, soybean production, low elevation, high hydrologic connectivity score, watersheds with currently high sediment and nutrient loads, near watershed outlet, and native Quercus/Carya species.

Table 1 contains the input values to calculate the scenarios in the opportunity set. There are two prices for carbon: a market price[7] and a social cost of carbon[8]. These two values bracket a reasonable price range for sequestration of LMV carbon. Forest costs include land purchase or rental, if applicable, and timber establishment and maintenance costs. Land cost for Madison Parish range between USD 1,675/ha and USD 4,586/ha and between USD 4,700/ha and USD 7,384/ha for Tensas Parish (2011). We assume that 50% of the reforested land comes from each Parish and that the land is valued at the average market price in each parish. Data input include area converted to forest, conditional probability of timber harvest output and price, rotation period, indirect-use value, discount rates, and conditional probability of quantity produced for an ecosystem service. The temporal risk of a wildfire has a probability less than 0.01 annually in the region based on the 2016 Madison and Tensas Parishes Hazard Mitigation Plans (Stephenson Disaster Management Institute 2016a; Stephenson Disaster Management Institute 2016b). We assume that $p_s\geq0.99$ .

[7]. https://www.arb.ca.gov/cc/capandtrade/auction/november_2012/updated_nov_results.pdf.
[8]. https://www3.epa.gov/climatechange/Downloads/EPAactivities/social-cost-carbon.pdf.

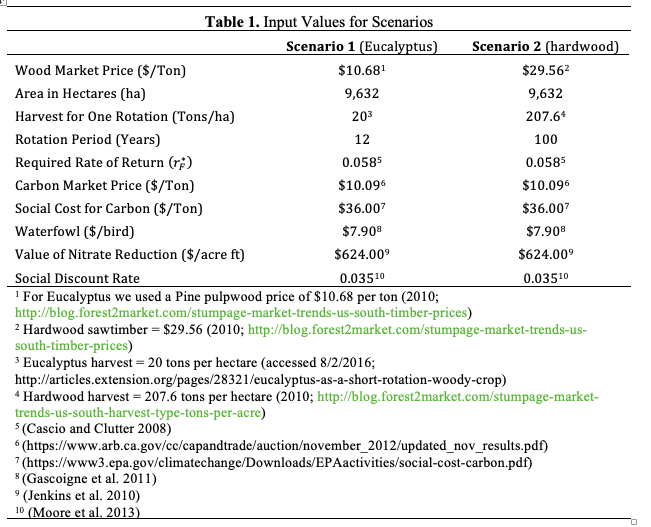
The indirect-use correlation matrix values $\rho_{B_{i\zeta k}}$ for the three ecosystem services are found in Table 2. These values are inputs to equation 8. They demonstrate that the integration of ecosystem services has both negative and positive correlations. Enhanced waterfowl habitat is negatively correlated with carbon sequestration, while improved water quality is positively correlated with carbon sequestration. Enhanced waterfowl habitat and improved water quality are negatively correlated, given their interaction with the carbon sequestration is expected.

IV. Results and Discussion

The forest outputs and market values for each scenario are listed in Table 3. The output of timber and wood products and the economic value of that production vary by s. Among the scenarios the benefits from the forest are greatest for the Eucalyptus scenarios. This outcome is the result of the multiple harvests from Eucalyptus species as compared to the single harvest for the hardwood scenarios. The differences among the scenarios can be seen in the first three lines of Table 3.

Adding in management costs decreases the value of the forest for the two Eucalyptus scenarios, however they still produce positive NRVs, while the hardwood forest is not economical. When land costs are included (last four lines of Table 3) all three scenarios are not economical. One way to increase an investor’s rate of return would be to include the ecosystem service values as a second asset to a forest security.

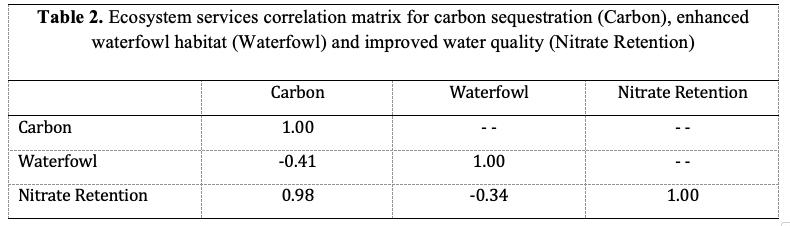
Turning to the ecosystem service outputs in Table 4, an interesting outcome from the calculations is the expected quantity change in the services provisioned relative to scenario 0. The scenarios provision considerable additionality for improvements in water quality relative to scenario 0. Scenario 1 has a greater impact than scenario 2, if only marginally. The additionality constraint can have a significant impact on portfolio choice. Only scenario 2 provisions a significant amount of additional waterfowl over the baseline. This result eliminates scenario 1 from the opportunity set if additionality to ecosystem services relative to the baseline is required.

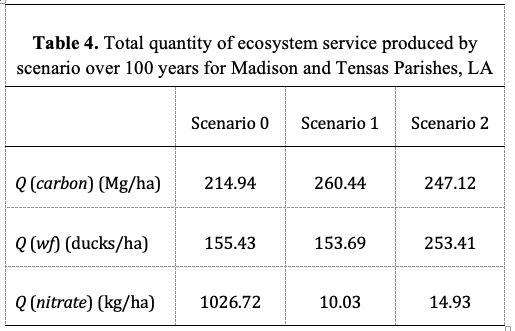
In Table 5, the economic benefits from ecosystem services are presented as an increase to social well-being that becomes part of the NRV. Here the results demonstrate a similar pattern as in Table 4. Carbon sequestration favors the Eucalyptus alternatives over the baseline and scenario 2 because of the Eucalyptus’s ability to sequester a great amount of carbon over a shorter time-period than the bottomland hardwood scenario. On the other hand, the opposite result occurs for the other two ecosystem services. As in Table 3, scenario 1 provides less value than the baseline, while for the other two ecosystem services scenario 2 is superior to the baseline and scenario 1.

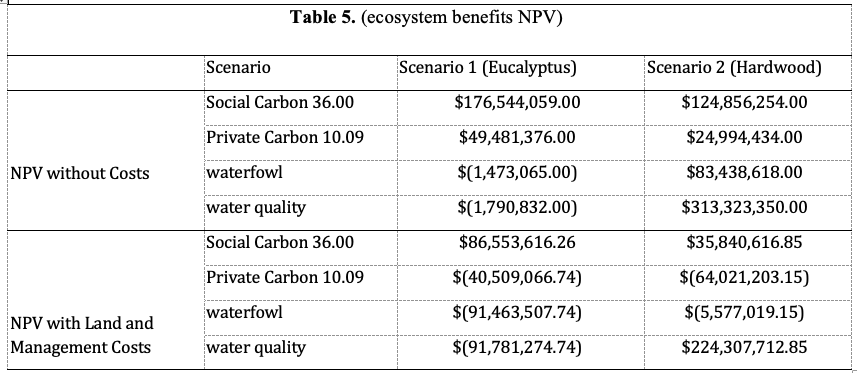
The expected net benefits for the scenarios for three ecosystem services are listed in Table 5. Further inspection of Table 5 reveals a wide range of expected benefits for the scenarios. For carbon sequestration, the Eucalyptus scenarios capture more carbon than the hardwood alternative. On the other hand, the distribution of the expected benefits for $wf$ and $nitrate$ in scenario 2 are greater than the expected benefits for scenario 1. This is because the hardwood alternative provisions greater quality and quantity of ecosystem services of $wf$ in the bottomlands and $nitrate$ removal in the water supply. The lack of benefits for scenario 1 is exacerbated by the shorter rotation cycle that leads to fewer services provisioned in a less mature forest. In addition, the Eucalyptus trees’ demand for water is greater than hardwoods’. Discounting increases these differences because the discount rate has a considerable impact in the later years of the forest lifetimes. Thus, investors can choose a bond for a bottomland hardwood forest that can be sold as timber and other wood products and that is worth more in the marketplace at the end of a rotation than a Eucalyptus forest. However, a Eucalyptus forest scenario could be chosen that possesses an excess return, $r\bar_s-r_F^\ast>0$ , that is equal to or greater than that of a hardwood forest (without indirect uses) if the positive carbon sequestration externality were included.

The correlation coefficient $\rho_{\upsilon B}$ is among the scenarios that include the baseline ranges between 0.84 and 0.97 (Lubowski et al. 2008). The estimated correlations in Lubowski et al. (2008) are based on a panel data model that estimates the probability of the conversion of agricultural land to forest. At this point all the necessary input components are accessible to calculate the $NRV$ and ${\bar{r}}_s$ for each $s$ .

Table 6 lists the $NRV$ and ${\bar{r}}_s$ for three distinct combinations of bottomland forest production and cost. They are (1) timber with up-front land costs and management costs, (2) ecosystem services with up-front land costs and management costs, (3) timber and ecosystem services with up-front land costs and management costs.

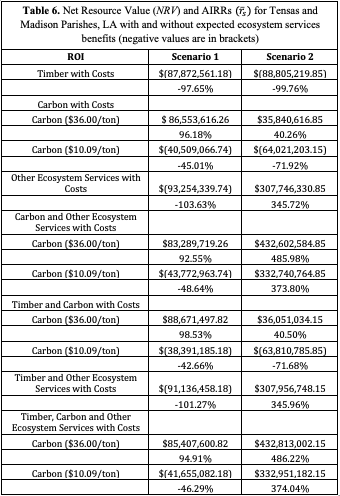
Each scenario and treatment listed in Table 6 reveals some interesting outcomes for $NRV$ and ${\bar{r}}_s$ . Both scenarios have negative $NRV$ s and ${\bar{r}}_s$ for forested products only because up-front land costs are much larger than wood revenue generated. Timber benefits and ecosystem service treatments with costs yield positive $NRV$ s and ${\bar{r}}_s$ for scenario 2, but a negative outcome for scenario 1. Scenario 2 for timber with up-front land and management costs yields the lowest $NRV$ and ${\bar{r}}_s$ .

Particular scenario treatments show that the AIRR would exceed the required return of 5.8% per year (Casio and Clutter 2008). Twenty-seven percent (6 / 22) of the development plans have an excess positive return because ${\bar{r}}_s-r_F^\ast>0$ , and according to the decision criterion are worth the investment. There are four more treatments that are close to the required return $r_F^\ast$ . The remainder of the opportunity set shows an AIRR improvement over the timber only treatments except for scenario 1, Timber and Other Ecosystem Services with Costs. This treatment shows that by not including carbon sequestration, the additional benefit from the other ecosystem services is small due to the short harvest rotation ${\bar{r}}_s-r_F^\ast=-1.01$ . For the most part, the scenario treatments that include the indirect-use asset create a greater Return on Investment. The best performer is scenario 2, Timber, Carbon and Other Ecosystem Services with Costs and Carbon (USD 36.00/ton). The combination for this treatment takes the greatest advantage of the long-term growth in value for both asset components, i.e., ${\bar{r}}_s-r_F^\ast=4.86$ . The addition of the indirect use asset increases returns and should be factored into the market price of a bond.

The means and standard deviations for the 2 scenarios and their treatments can be combined into a forest market with varying attributes over the opportunity set. The mean is an average of the expected returns from Table 6 while the standard deviation is calculated using the mean and calculating the sum of the squared deviations from this mean. The forest security opportunity set has an AIRR, median and standard deviation of -102.98%, -9.36% and 0.8862 for scenario 1 and 2249.12%, 204.47% and 2.356 for scenario 2.

Depending on investor preferences the proportion of a bond’s direct and indirect-use components will vary. For example, an investor with a preference for a short rotation forest with the intent to maximize timber market products may favor a monoculture forest like Eucalyptus (scenario 1), which produce fewer and / or lower valued ecosystem services. In this case the weights of the assets in the security are skewed more toward market outputs. For example, a good investment hedge would be a Eucalyptus plantation with a 12-year rotation period (variations of scenarios 1). On the other hand, an investor who prefers greater amounts of ecosystem services could emphasize the indirect-use component so that the rotation could be as long as 100 years or in perpetuity. In this case a bottomland hardwood forest would be chosen because of the greater value of ecosystem services that would be produced over the longer rotation time (scenario 2).

The market prices for Tensas and Madison Parish, LA forest zero-coupon bonds can be found in Table 7 and are based on the AIRR for each treatment that has a positive NRV in Table 6. Current zero-coupon bond market prices are based on equation 11 and listed in Table 7 as offers in thousand dollar increments over a thirty-year period.

Entries in Table 7 include 12 treatments that are underpriced and 10 treatments that are over-priced. The 12 underpriced bonds have current market prices that vary over a wide range from USD 332.33 to USD 886.44. The 10 over-priced treatments current prices range from USD 1,157 to USD 1357.13. Table 7 shows a large current price discount that occurs when indirect uses are added.

Market prices for Tensas and Madison Parish forest with an annual coupon for a traditional bond (equation 12) are listed in Table 8. Bond pricing assumes Par and Redemption are the same with coupon payments (5.8%) over 30 years offered in thousand dollar increments. Bonds that sell above par are due to the fact that the AIRR (Table 6) is below the coupon rate, which makes the bond attractive. If the bond sells below par it is because the AIRR is above the market rate (coupon of 5.8%).

Table 8 displays the same trend as Table 7. The market price can vary widely among the scenarios, and even negative returns occur. The current price for a traditional bond is higher due to the yearly coupon rate. Entries in Table 8 are similar to Table 7 with 12 treatments that are underpriced and 10 treatments that are over-priced. Both scenarios have alternatives that pay a yearly return, which is above the market rate making them more attractive to the investor.

A zero-coupon sustainable forestry bond for many of the scenarios is sold at a low price due to the large AIRR’s from the ecosystem services. However, if these bonds are oversubscribed (i.e. have increased demand) the price could rise over the original price, which could be a benefit for the seller of the bond as it lowers the return that must be paid. A traditional sustainable forestry bond sells above par in all cases due to the coupon rate of 5.8% being above the market rate of return (the 100-year AIRR). Because these bonds provide a yearly payout (i.e. coupon) they could be viewed as a safer alternative, but this depends upon investor preferences.

In addition to the type of bond offered, other factors influence the outcomes, such as length of rotation, discounting, harvest and perpetuity values, indirect uses, and land cost. Including carbon sequestration benefits and land acquisition cost has a major impact on the investment choice. If the objective is to sequester carbon emissions a eucalyptus forest is the best alternative. On the other hand, if the objective is to maximize net benefits from other ecosystem services (i.e. waterfowl and nitrate retention) a hardwood forest is the best alternative.

Currently, a sustainable forestry bond includes the nonmarket effects of ecosystem services without wood products and a traditional forest bond includes wood products without ecosystem services. If the positive ecosystem services are not internalized into the calculated AIRR and subsequently not employed in calculating the price of a bond, returns from the wood products are negative. Alternatively, if ecosystem services are packaged as a separate asset and not included with the wood products, the returns, while positive (Table 6), do not account for the true value of the forest. As shown in Table 6 the best return is found when timber and ecosystem services are combined. Combining these two benefits into one asset internalizes the positive externalities of reforestation.

V. Summary and Avenues for Future Research

As ecosystem service markets thicken a sustainable forestry bond that can be traded is realizable in a market that incorporates direct use—harvestable timber—and indirect use—ecosystem services (Boyd et al. 2015). An investor in this type of forest market requires the quantification of the expected return and risk among ecosystem service assets and between direct and indirect-use land portfolios. The concept of a market-based approach to long-term forest investments has been proposed as an investment security (Boyd and Epanchin-Niell 2017; Górriz-Mifsud et al. 2016; Binkley et al. 2006). However, a green bond for a market driven good such as timber does not produce a worthwhile return at current wood product market prices. Adding in the ecosystem service benefits does create a positive return, however the objective function is important in determining the size of the return.

Here we have developed a sustainable forestry bond that characterizes the investment as separable portfolios of direct and indirect uses that are integrated into one market product. The sustainable forestry bond demonstrates that a spatial portfolio model can be used to facilitate market functions and transactions. Investors expect a return of a specific sustainable forestry bond to exceed the required return, which affects what the investor is willing to pay for the security. The difference between ${\bar{r}}_s$ and $r_F^\ast$ is used as an investment decision criterion.

A second use of the decision criterion is that it represents the degree to which a specific forest security is mispriced (Sharpe et al. 1999). This guideline is one way to assess whether a particular forest security is undervalued or overvalued and by how much.

Including the ecosystem services ${\bar{r}}_{B_s}$ does impact the total return of the security positively. Our results demonstrate that the benefits of ecosystem services are significant in the investment decision for the scenarios. The sustainable forestry bond is undervalued when $P_{F\left(s\right)}$ is less than $Pn$ : or, if the expected return is less than the required rate of return, the forest security would be overvalued because $P_{F\left(s\right)}$ is higher than $Pn$ . Some interesting differences are apparent in a comparison of Tables 7 and 8. In either case, the sustainable forestry bond shows that adding ecosystem services is a plus for renewable resources markets. The multiple harvest nature of Eucalyptus in scenario 1 leads to a bond valuation that is near par at the higher social cost of carbon versus the lower cost. These features allow the market to include choices for the interest rate and forest rotation for timber harvest that can differ for the social time preference for ecological resources. Investors can make individual choices for weighting the security with more (less) direct or less (more) indirect uses. Adding the indirect-use asset is an attraction to investors because it allows an assessment of the wealth advantage that can be gained by internalizing the ecological assets (environmental externalities) into the security. Furthermore, the current prices for the zero-coupon and traditional bonds (Tables 7 and 8) reflect this outcome.

Adding the indirect-use asset produces an increase in the cash flow to the forest bond, which affects the Par value, and consequently the Return on Investment.In this analysis we assume that ecosystem services are time invariant, this may not be the case as a loss or benefit in ecosystem services produces a perpetual loss or benefit. Future research should investigate this arena and how it impacts market valuation through time.

Appendix: Decomposition of Ecosystem Services Production and Benefits

Conversion of land from a current land use such as agriculture to a forest includes changes in the values associated with both the direct and indirect uses of the land. Besides the change from a row crop to trees, it can be expected that a conversion will increase the sequestered carbon, reduce agrochemicals in surface and groundwater, and improve natural habitats for bird species (Nelson et al. 2009). Estimation of the benefits of these ecosystem services in equation 4 is based on landscapes that have multiple land-use, land-cover (LULC) classes, each with a range of biophysical characteristics and corresponding ecosystem services:

$B_k=P_i\times\ Q_k$

where $B_k\sum_{t=0}^{n}\sum_{k=1}^{K}\sum_{i=1}^{I}b_{ikt}$ , $bikt$ of ecosystem service $i,\ i=0,\ldots,I$ , produced in $k$ grid cells at time $t,t=0,\ldots,n$ years, $P_i=\sum_{i=1}^{I}p_i$ , $pi$ is the price or indirect-use value of ecosystem $i$ , $Q_k=\sum_{t=0}^{n}\sum_{k=1}^{K}\sum_{i=1}^{I}q_{ikt}$ , $Qk$ is the total quantity of ecosystem services produced over the rotation period of $t$ , $qikt$ is the quantity of ecosystem service produced over time $t$ in $k$ cells and is based on the forest type and growth rate (http://www.sfrc.ufl.edu/extension/florida_forestry_information/forest_management/growth_and_yield.html accessed 5/31/16). Forest indirect-use values pi for each service exist (Bennett and Carroll 2014)[9] and are traded currently (Forest Trends’ Ecosystem Marketplace 2015, http://www.ecosystemmarketplace.com/wp-content/uploads/2016/01/Ecosystem-Marketplace-Market-Primer-2015-Final.pdf, accessed 5/9/16).

[9]. http://www.ecosystemmarketplace.com/marketwatch/carbon/north-america/.
http://www.watershedconnect.org/programs/index.php.
http://www.ecosystemmarketplace.com/marketwatch/biodiversity/north-america/.

A forest scenario provides some or all the possible ecosystem services available in a forest as the benefits for the measured ecosystem services present and is denoted B_k. To compare the benefit of indirect uses for forest ecosystem services to an existing land use is to find:

$P_i\Delta Q_k$ (A1)

The indirect-use value for the forest is:

$B_k=P_i\Delta Q_k=P_i\left(Q_k-Q_0\right)$ (A2)

where $B_{k\ \ }$ are the ecosystem services benefits, which is the difference between $B_{k\ \ }$ with development and the ecosystems services benefits for the current land use, that is, the baseline, $\ \Delta Q_{ik}$ is the change in quantity of ecosystem service $i$ in $k$ cells produced with development $Q_{ik}$ , and $Q_0$ is the quantity of an ecosystem service produced in the baseline. The baseline produces interdependent ecosystem services (Sauer and Wossink 2013). $B_{k\ \ }$ becomes the input to a land use security that can represent an indirect-use cash flow.

Biographies

Richard L. Bernknopf is a research professor in the Department of Economics at the University of New Mexico and a Nonresident Research Fellow at Resources for the Future in Washington, DC. He is the director of the Science Impact Laboratory for Policy and Economics at the University of New Mexico. Before joining the faculty at UNM, Dr. Bernknopf was an economist with the United States Geological Survey for more than thirty-eight years. Dr. Bernknopf’s research focuses on the demonstration of the economic value of natural science and earth observation and the translation of that data into information compatible with decision-making processes. Professor Bernknopf can be reached at rbern[at]unm[dot]edu.

Craig D. Broadbent is a professor of Economics at Brigham Young University – Idaho. He is a trained environmental and resource economist with specialties in water resource markets and the valuation of non-market environmental goods and services. He has published articles on water markets in Environmental and Resource Economics; Water Economics and Policy; and Agricultural Water Management. In investigating non-market valuation techniques, he has published articles in the Journal of Environmental Planning and Management; Ecohydrology; and Social Sciences. Professor Broadbent can be reached at broadbentcr[at]byui[dot]edu.

References

Barnett, A., J. Fargione, and M. Smith. 2016. “Mapping Trade-Offs in Ecosystem Services from Restoration in the Mississippi Alluvial Valley.” Bioscience 66:223–237. Available from https://doi.org/10.1093/biosci/biv181 or http://bioscience.oxfordjournals.org/.

Bass, R., P. Murphy, and H. Dithrich. 2019. Scaling Impact Investment in Forestry. New York, NY: Global Impact Investing Network. Available from https://thegiin.org/research/publication/forestry.

Benítez, P., I. McCallumb, M. Obersteiner, and Y. Yamagata. 2007. “Global Potential for Carbon Sequestration: Geographical Distribution, Country Risk and Policy Implications.” Ecological Economics 60:572–583.

Benítez, P., and M. Obersteiner. 2006. “Site Identification for Carbon Sequestration in Latin America: A Grid-based Economic Approach.” Forest Policy and Economics 8:636–651.

Bennett, G., and N. Carroll. 2014. Gaining Depth: State of Watershed Investment 2014. Available online from www.ecosystemmarketplace.com/reports/sowi2014.

Bennett, G., N. Carroll, K. Sever, A. Neale, and C. Hartley. 2016. An Atlas of Ecosystem Markets in the United States. Washington, DC.: Forest Trends’ Ecosystem Marketplace. Accessed December 22, 2016 http://forest-trends.org/releases/p/atlas_of_ecosystem_markets.

Bernknopf, R., C. Broadbent, D. Adhikari, S. Mamun, V. Tidwell, C. Babis, and E. Pindilli. 2019. Multi-resource Analysis— A Proof of Concept Study of Natural Resource Tradeoffs in the Piceance Basin, Colorado, Using the Net Resources Assessment (NetRA) Decision Support Tool. U.S. Geological Survey Scientific Investigations Report 2019–5086. Available from https://doi.org/10.3133/sir20195086.

Binkley, C., S. Beebe, D. New, and B. von Hagen. 2006. “An Ecosystem-based Forest Investment Strategy for the Coastal Temperate Rainforest of North America (GreenWood Resources Research Note 2006-1).” Portland, OR: GreenWood Resources. Available from http://greenwoodresources.com/wp-content/uploads/2014/06/AnEcosystemBasedForestryInvestent.pdf.

Bond, C., A. Strong, N. Burger, S. Weilant, U. Saya, and A. Chandra. 2017. Resilience Dividend Valuation Model: Framework Development and Initial Case Studies. Santa Monica, CA: RAND Corporation. ISBN: 978-0-8330-9896-2.

Boyd, J., and S. Banzhaf. 2007. “What Are Ecosystem Services? The Need for Standardized |Environmental Accounting Units.” Ecological Economics 63:616–626.

Boyd, J., R. Epanchin-Niell, and Juha Siikam ki. 2012. “Conservation Return on Investment Analysis: A Review of Results, Methods, and New Directions.” RFF DP 12-01, Washington, DC: Resources for the Future.

Boyd, J., R. Epanchin-Niell, and Juha Siikam ki. 2015. “Conservation Planning: A Review of Return on Investment Analysis.” Review of Environmental Economics and Policy 9: 23–42. https://doi.org/doi:10.1093/reep/reu014.

Burtraw, D. 2016. “Commentary: Why California’s Cap-and-Trade Program Works.” Resources no. 193 (Fall).

Business Dictionary (2020a). Available from http://www.businessdictionary.com/definition/zero-coupon-bond.html.

Business Dictionary (2020b). Available from http://www.businessdictionary.com/definition/coupon-bond.html.

Cascio, A., and M. Clutter. 2008. “Risk and Required Return Assessments of Equity Timberland Investments in the United States.” Forest Products Journal 58:61–70.

Chivoiu, B., S. Faulkner, J. Quansah, W. Barrow, and H. Waddle, et al. 2010. “Integrated Modeling Framework for Forecasting Ecosystem Services.” Paper presented at ACES: A Community on Ecosystem Services. Available from www.conference.ifas.ufl.edu/aces or https://conference.ifas.ufl.edu/aces10/Abstract_BOOK.pdf.

Convertino, M., and L. Valverde. 2013. “Portfolio Decision Snalysis Framework for Value-focused Ecosystem Management.” PLOS ONE 8:e65056. Available from https://doi.org/10.1371/journal.pone.0065056.

Cubbage, F., P. MacDonagh, G. Balmelli, V. Olmos, A. Bussoni, R. Rubilar, R. De La Torre, R. Lord, J. Huang, V. Alfonzo Hoeflich, M. Murara, B. Kanieski, P. Hall, R. Yao, P. Adams, H. Kotze, E. Monges, C. Hernández Pérez, J. Wikle, R. Abt, R. Gonzalez, and O. Carrero. 2014. “Global Timber Investments and Trends, 2005–2011.” New Zealand Journal of Forestry Science 44 (Suppl 1): S7.

Edwards, S., J. Link, and B. Rountree. 2004. “Portfolio Management of Wild Fish Stocks.” Ecological Economics 49:317–329.

Erhart, S. 2018. “Exchange-Traded Green Bonds.” Discussion paper. Journal of Environmental Investing (Oct. 9): 1–40. Available from https://www.thejei.com/exchange-traded-green-bonds-by-szilard-erhart/.

Faulkner, S., W. Barrow Jr, B. Keeland, S. Walls, and D. Telesco. 2011. Effects of Conservation Practices on Wetland Ecosystem in the Mississippi Alluvial Valley. Supplement: Conservation of Wetlands in Agricultural Landscapes. Ecological Applications 21, no. 3: S31–S48. Available from https://www.jstor.org/stable/23021649.

Fenichel, E., and J. Abbott. 2014. “Natural Capital: From Metaphor to Measurement. Journal of the Association of Environmental and Resource Economists 1:1–27. Accessed July 26, 2018, from http://dx.doi.org/10.1086/676034.

Florida Forest Stewardship. 2010. “Growth and Yield.” Florida Forest Stewardship. University of Florida IFAS Extension. Available from http://www.sfrc.ufl.edu/Extension/florida_forestry_information/forest_management/growth_and_yield.html.

Forest Trends’ Ecosystem Marketplace. 2016. An Atlas of Ecosystem Markets in the United States. Washington, DC: Forest Trends’ Ecosystem Marketplace. Available from info@ecosystemmarketplace.com.

Frey, G., D. E. Mercer, F. Cubbage, and R. Abt. 2013. “A Real Options Model to Assess the Role of Flexibility in Forestry and Agroforestry Adoption and Disadoption in the Lower Mississippi Alluvial Valley.” Agricultural Economics 44:73–91.

Gascoigne, W., D. Hoag, L. Koontz, B. Tangen, T. Shaffer, and R. Gleason. 2011. “Valuing Ecosystem and Economic Services across Land-Use Scenarios in the Prairie Pothole Region of the Dakotas, USA.” Ecological Economics 70:1715–1725. Available from https://doi.org/10.1016/j.ecolecon.2011.04.010.

Górriz-Mifsud, E., E. Varela, M. Piqué, and I. Prokofieva. 2016. “Demand and Supply of Ecosystem Services in a Mediterranean Forest: Computing Payment Boundaries.” Ecosystem Services 17:53–63. Available from http://dx.doi.org/10.1016/j.ecoser.2015.11.006.

Goulder, L. 2013. “Markets for Pollution Allowances: What Are the (New) Lessons?” Journal of Economic Perspectives 27:87–102.

Hamrick, K. 2016. State of Private Investment in Conservation 2016: A Landscape Assessment of an Emerging Market. Washington, DC: Forest Trends’ Ecosystem Marketplace. Available from https://www.forest-trends.org/publications/state-of-private-investment-in-conservation-2016/.

Heal, G., E. Barbier, K. Boyle, A. Covich, S. Gloss, C. Hershner, J. Hoehn, C. Pringle, S. Polasky, K. Segerson, K. Shrader-Frechette, et al.; the National Research Council. 2005. Valuing Ecosystem Services: Toward Better Environmental Decision-Making. Washington, DC: The National Academies Press.

Healy, T., T. Corriero, and R. Rozenov. 2005. “Timber as an Institutional Investment.” The Journal of Alternative Investments 8, no. 3 (Winter): 60–74.

Herbohn, K., and S. Henderson. 2002. “Forestry Managers’ Perceptions of the Feasibility of Incorporating Non-Market Value Estimates into Financial Environmental Reporting.” Economic Analysis & Policy 32, Special Issue: 131–153.

Holling, C., and L. Gunderson. 2002. “Resilience and Adaptive Cycles.” In Panarchy: Understanding Transformations in Human and Natural Systems, edited by C. S. Holling and Lance H. Gunderson, 25–62. Washington, DC: Island Press.

Ingram, B., and F. Malamud-Roam. 2015. The West without Water: What Past Floods, Droughts, and Other Climatic Clues Tell Us about Tomorrow. Berkeley and Los Angeles, CA: University of California Press.

Jenkins, W., B. Murray, R. Kramer, and S. Faulkner. 2010. “Valuing Ecosystem Services from Wetlands Restoration in the Mississippi Alluvial Valley.” Ecological Economics 69:1051–1061. Available from https://doi.org/10.1016/j.ecolecon.2009.11.022; or https://pubs.er.usgs.gov/publication/70037120.

Kirilenko, A., B. Chivoiu, J. Crick, A. Ross-Davis, K. Schaaf, G. Shao, V. Singhania, and R. Swihart. 2007. “An Internet-Based Decision Support Tool for Non-Industrial Private Forest Landowners.” Environmental Modelling & Software 22:1498–1508.

Laffont, J-J. 1989. The Economics of Uncertainty and Information. Translated by John P. Bonin and Hélène Bonin. Cambridge, MA: MIT Press.

Lange, G-M. 2004. “Manual for Environmental and Economic Accounts for Forestry: A Tool for Cross-Sectoral Policy Analysis.” Working Paper, Food and Agricultural Organization of the United Nations. Available from ftp://ftp.fao.org/docrep
/fao/007/j1972e/j1972e00.pdf; or http://www.fao.org/3/j1972e/j1972e00.htm; or http://www.fao.org/tempref/docrep/fao/007/j1972e/j1972e00.pdf.

Lower Mississippi Valley Joint Venture. 2002. Physiography of the Mississippi Alluvial Valley. Migratory Bird Science Team – Technical Note #2.

Lubowski, R., A. Plantinga, and R. Stavins. 2008. “What Drives Land-Use Change in the United States? A National Analysis of Landowner Decisions.” Land Economics 84:529–550.

Madeira, L. 2016. The Forest Resilience Bond. Katoomba Marketplace: Investment Opportunities in Green Infrastructure, Blue Forest Conservation presentation, October 11, 2016. Available from https://www.forest-trends.org/wp-content/uploads/2017/11/Madeira_Forest-Resilience-Bond.pdf.

Magni, C. 2010. “Average Internal Rate of Return and Investment Decisions: A New Perspective.” The Engineering Economist 55:150–180. Available from https://doi.org/10.1080/00137911003791856.

Marchioni, A., and C. Magni. 2018. “Investment Decisions and Sensitivity Analysis: NPV-Consistency of Rates of Return.” European Journal of Operational Research 268:361–472.

Mei, B., and M. Clutter. 2010. “Evaluating the Financial Performance of Timberland Investments in the United States.” Forest Science 56:421–428.

Moore, R., T. Williams, E. Rodriguez, and J. Hepinstall-Cymerman. 2013. “Using Nonmarket Valuation to Target Conservation Payments: An Example Involving Georgia’s Private Forests.” Journal of Forestry 111:261–270.

Murdoch, W., S. Polasky, K. Wilson, H. Possingham, P. Kareiva, and R. Shaw. 2007. “Maximizing Return on Investment in Conservation.” Biological Conservation 139:375–388. Available from https://doi.org/10.1016/j.biocon.2007.07.011.

Nelson, E., G. Mendoza, J. Regetz, S. Polasky, H. Tallis, D. R. Cameron, K. M. A. Chan, G. Daily, J. Goldstein, P. Kareiva, E. Lonsdorf, R. Naidoo, T. Ricketts, and M. R. Shaw. 2009. “Modeling Multiple Ecosystem Services, Biodiversity Conservation, Commodity Production, and Tradeoffs at Landscape Scales.” Frontiers in Ecology and the Environment 7:4–11. Available from https://doi.org/10.1890/080023.

Pindilli, E., and F. Casey. 2015. Biodiversity and Habitat Markets—Policy, Economic, and Ecological Implications of Market-Based Conservation. U.S. Geological Survey Circular 1414. Reston, VA: U.S. Department of the Interior, U.S. Geological Survey. Available from https://doi.org/10.3133/cir1414.

Raudsepp-Hearne, C., G. Peterson, and E. Bennett. 2010. “Ecosystem Service Bundles for Analyzing Tradeoffs in Diverse Landscapes.” PNAS, 107: 5242–5247. Available from www.pnas.org/cgi/doi/10.1073/pnas.0907284107.

Redmond, C., and F. Cubbage. 1988. “Portfolio Risk and Returns from Timber Asset Investments.” Land Economics 64:325–337. Accessed January 28, 2015 from http://www.jstor.org/stable/3146305.

Rinaldi, F., and R. Jonsson. 2013. “Risks, Information and Short-Run Timber Supply.” Joint Research Centre, European Commission (JRC). Forests 4 (4):1158–1170. Available from https://doi.org/10.3390/f4041158.

Sanchirico, J., M. Smith, and D. Lipton. 2008. “An Empirical Approach to Eecosystem-Based Fishery Management.” Ecological Economics 64:586–596.

Sauer, J., and A. Wossink. 2013. “Marketed Outputs and Non-Marketed Ecosystem Services: The Evaluation of Marginal Costs.” European Review of Agricultural Economics 40:573–603. Available from https://doi.org/10.1093/erae/jbs040; or http://erae.oxfordjournals.org/.

Seaber, P., F. Kapinos, and G. Knapp. 1994. “Hydrologic Unit Maps.” U.S. Geological Survey Water-Supply Paper 2294. Denver, CO: U.S. Geological Survey.

Sharpe, W., G. Alexander, and J. Bailey. 1999. Investments. 6th ed. Upper Saddle River, NJ: Prentice Hall.

Sigman, K. 2005. “Portfolio Mean and Variance.” Available from http://www.columbia.edu/~ks20/FE-Notes/4700-07-Notes-portfolio-I.pdf.

Stephenson Disaster Management Institute (SDMI). 2016a. Madison Parish Hazard Mitigation Plan Update. Baton Rouge, LA: Louisiana State University – Business Education Complex. Available from https://hmplans.sdmi.lsu.edu/Home/Parish/madison.

Stephenson Disaster Management Institute (SDMI). 2016b. Tensas Parish Hazard Mitigation Plan Update. Baton Rouge, LA: Louisiana State University – Business Education Complex. Available from https://hmplans.sdmi.lsu.edu/Home/Parish/tensas.

Straka, T., S. Bullard, and M. Dubois. 2001. “Introduction to Forestry Investment Analysis: Part I. Basic Investment Characteristics and Financial Criteria.” Faculty Publications, Paper 91. Available from http://scholarworks.sfasu.edu/forestry/91.

Sun, C., and D. Zhang. 2001. “Assessing the Financial Performance of Forestry-Related Investment Vehicles: Capital Asset Pricing Model vs. Arbitrage Pricing Theory.” American Journal of Agricultural Economics 83:617–628.

U.S. Geological Survey. 1999. “Hydrologic Units.” U.S. Geological Survey Information Sheet, Reston, VA: USGS.

van Kooten, G., and E. Bulte. 2000. The Economics of Nature: Managing Biological Assets. Oxford: Blackwell ISBN: 0 6312 1895 5.

Velloso, H., et al., Economic Commission for Latin America and the Caribbean (ECLAC). 2017. The Rise of Green Bonds: Financing for Development in Latin America and the Caribbean. Washington, DC: ECLAC; United Nations publication LC/WAS/TS.2017/6.

Washburn, C., and C. Binkley.1989. “Some Problems in Estimating the Capital Asset Pricing Model for Timberland Investments.” In Proceedings of the 1989 Southern Forest Economics Workshop.

Yao, W., B. Cheng, and B. Mei. 2016. “Investor Sentiment and Timberland Investment Returns.” Forest Products Journal 66:147–154. Available from http://dx.doi.org/10.13073/FPJ-D-15-00013.

Post Views: 4,276

Estimating Forest Sustainability Bond Prices for Natural Resource and Ecosystem Services Markets

Most Viewed Articles

Categories

Share this article

FOR CONTRIBUTORS

FOR SPONSORS

Estimating Forest Sustainability Bond Prices for Natural Resource and Ecosystem Services Markets

Most Viewed Articles

Tags

Categories

Share this article